Authors:
(1) Gopal Yadav, Department of Physics, Indian Institute of Technology & Chennai Mathematical Institute.
Table of Links
Brief Review of Wedge Holography
Emerging Multiverse from Wedge Holography
Application to Information Paradox
Application to Grandfather Paradox
Acknowledgements and References
4 Application to Information Paradox


• Boundary Description: BCFT is living at the AdSd+1 boundary with (d−1)-dimensional boundary.
• Intermediate Description: 2n gravitating systems interact with each other via transparent boundary conditions at the (d − 1)-dimensional defect.
• Bulk Description: Gravity dual of BCFT is Einstein gravity in the bulk. Consistency Check: Let us check the formula given in (22) for n = 2.
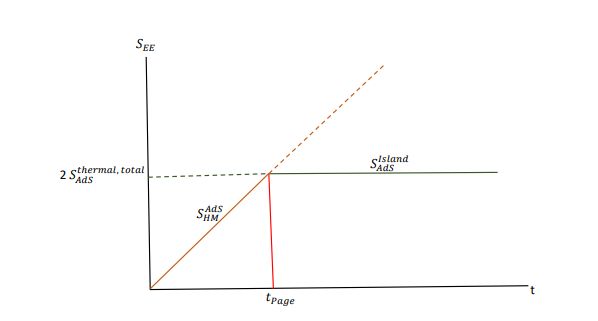
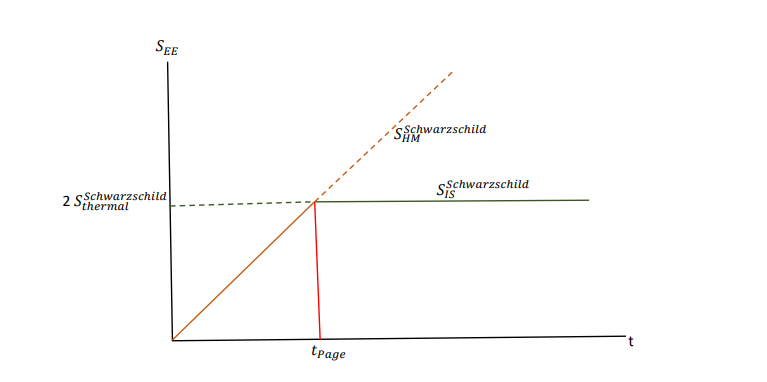
4.1 Page Curve of Eternal AdS Black Holes in n = 2 Multiverse
First, we will calculate the thermal entropies of black holes. The metric of the black holes in AdS background is:

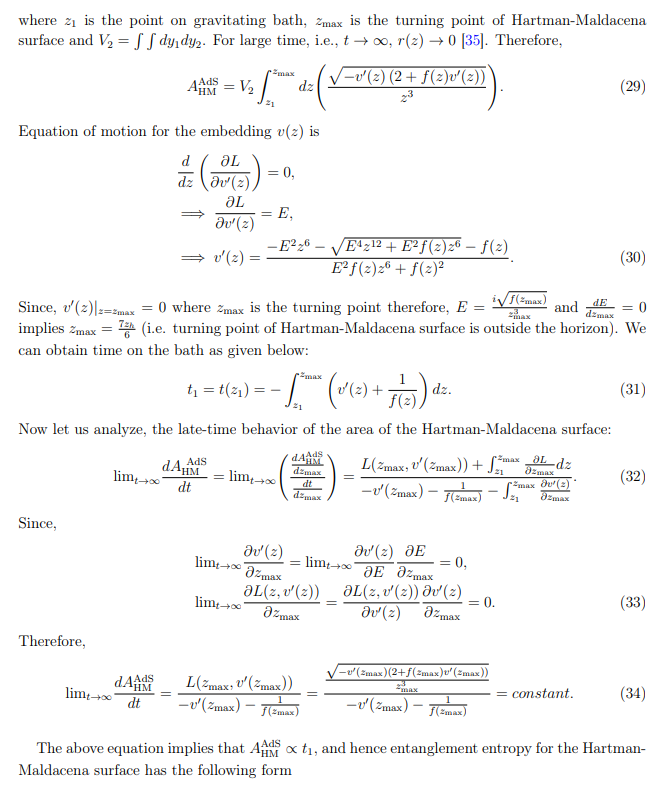
This corresponds to an infinite amount of Hawking radiation when t1 → ∞, i.e., at late times, and hence leads to information paradox.
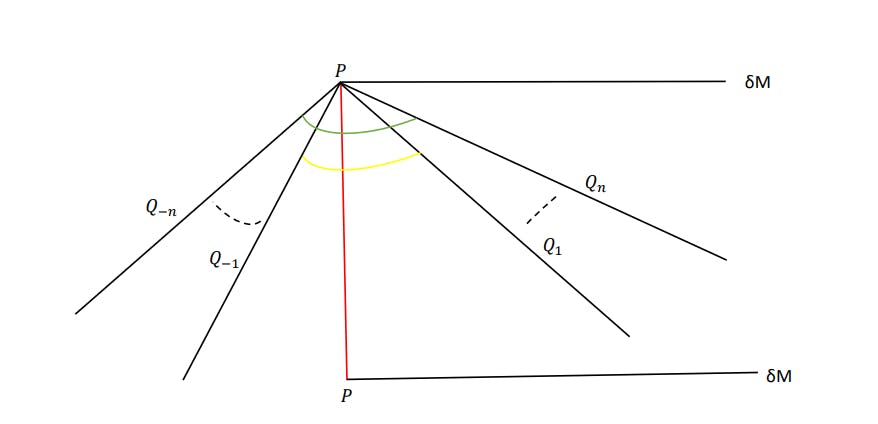
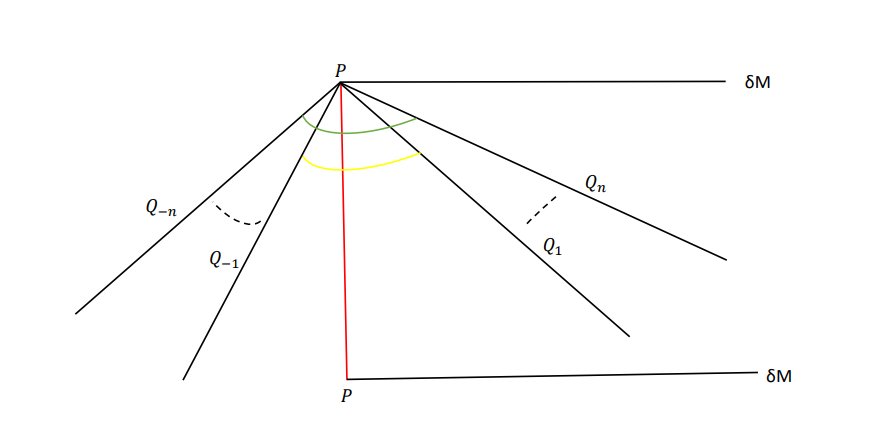
Entanglement entropy contribution from Island surfaces: Now consider the island surfaces parametrize as t = constant and z ≡ z(r). Entanglement entropy of two eternal AdS black holes for the island surfaces can be obtained using (22). Since there are two island surfaces(I1 and I2) stretching between the Karch-Randall branes located at r = ±ρ (I1) and r = ±2ρ (I2), and hence we can write (22) for the same as given below
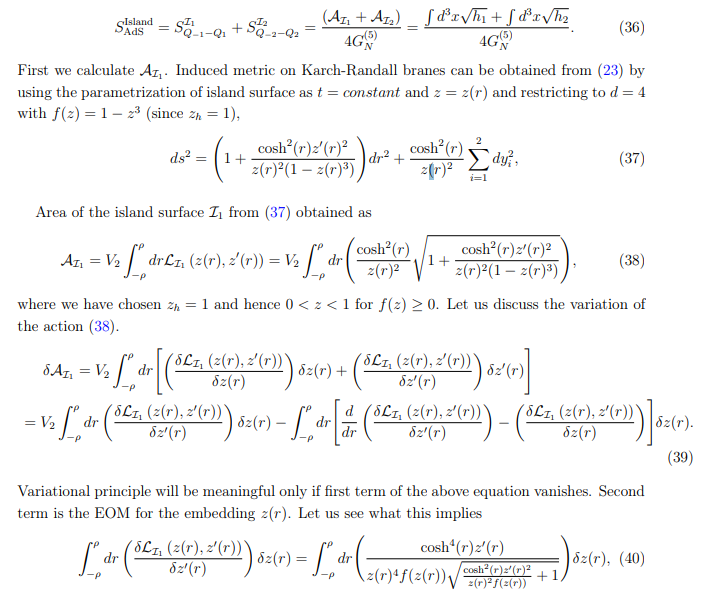

4.2 Page Curve of Schwarzschild de-Sitter Black Hole
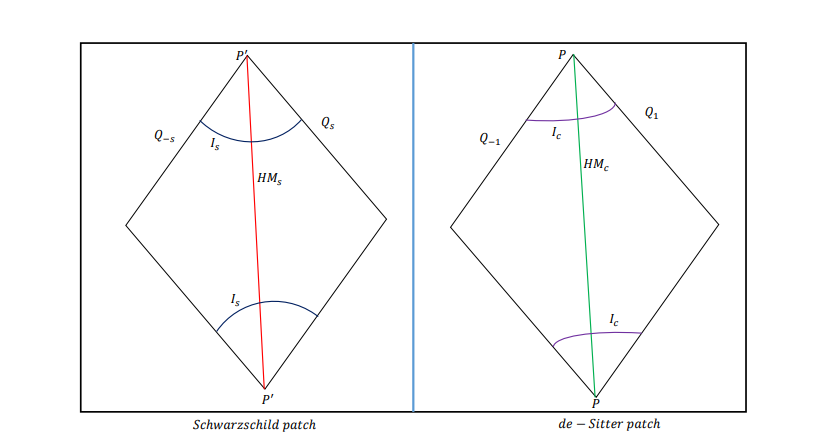
In this section, we study the information paradox of the Schwarzschild de-Sitter black hole. As discussed in section 3.3, we can not have mismatched branes connected at the same defect. Therefore, we study this problem in two parts by first calculating the Page curve of the Schwarzschild patch and then the Page curve of the de-Sitter patch similar to the non-holographic model [58]. This can be done as follows. We study the Schwarzschild patch in subsection 4.2.1 where we consider two flat space branes embedded in the bulk and de-Sitter patch in subsection 4.2.2 with two de-Sitter branes. We have shown the setup in Fig. 8. The setup is two copies of wedge holography with flat space and de-Sitter branes in Schwarzschild and de-Sitter patches respectively.
4.2.1 Schwarzschild patch
Since for Schwarzschild black hole, Λ = 0, therefore to realize Schwarzschild black hole on KarchRandall brane, we need to consider the flat space black holes. It was shown in [42] that one can get flat space black holes on Karch-Randall branes provided bulk metric should have the following form:
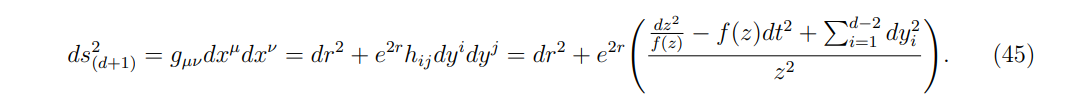
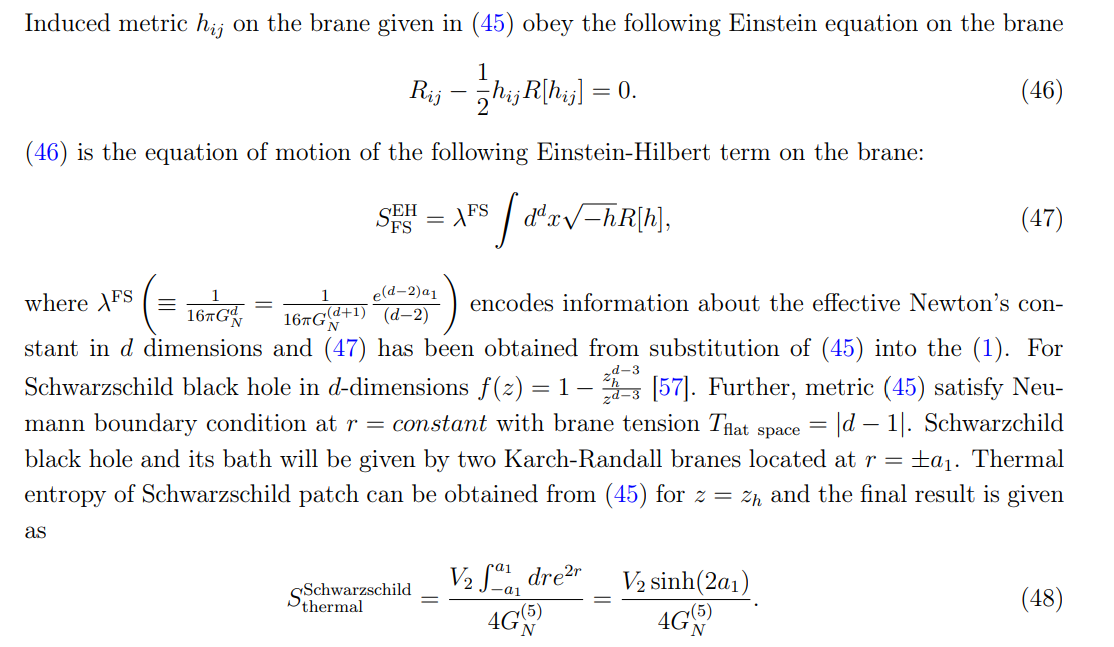


4.2.2 de-Sitter patch
de-Sitter black hole and its bath will be located at r = ±ρ. The Metric for the bulk which contains de-Sitter branes is
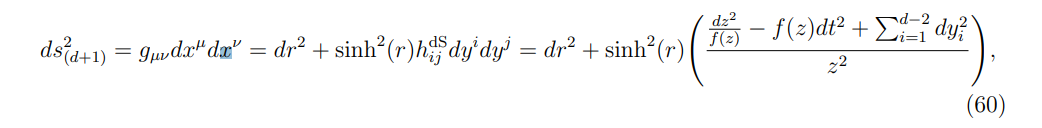

(65) Substituting v 0 (z) from (64) into (65) and using f(z) = 1 − z 2 , we set zs = 1 for simplification, EOM (65) simplifies to the follow
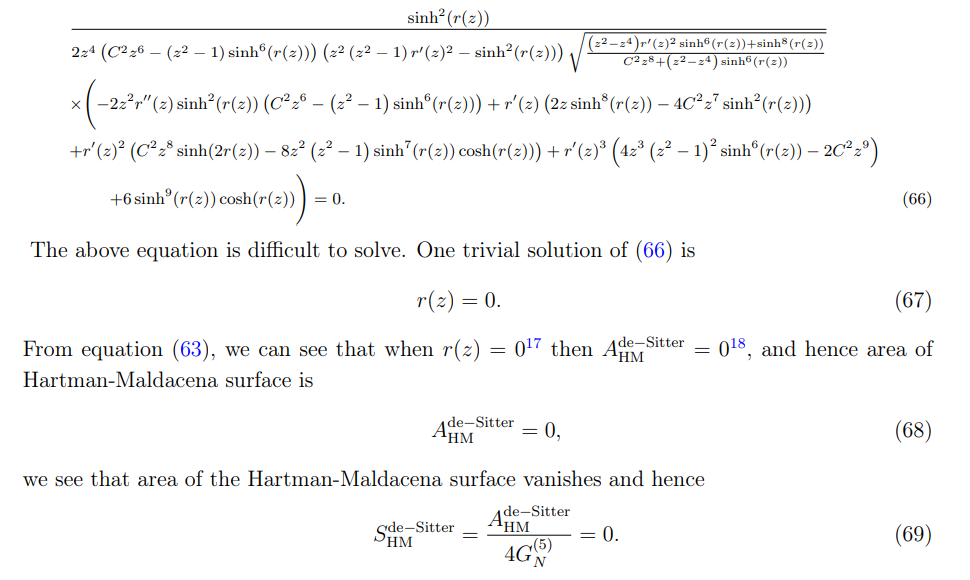
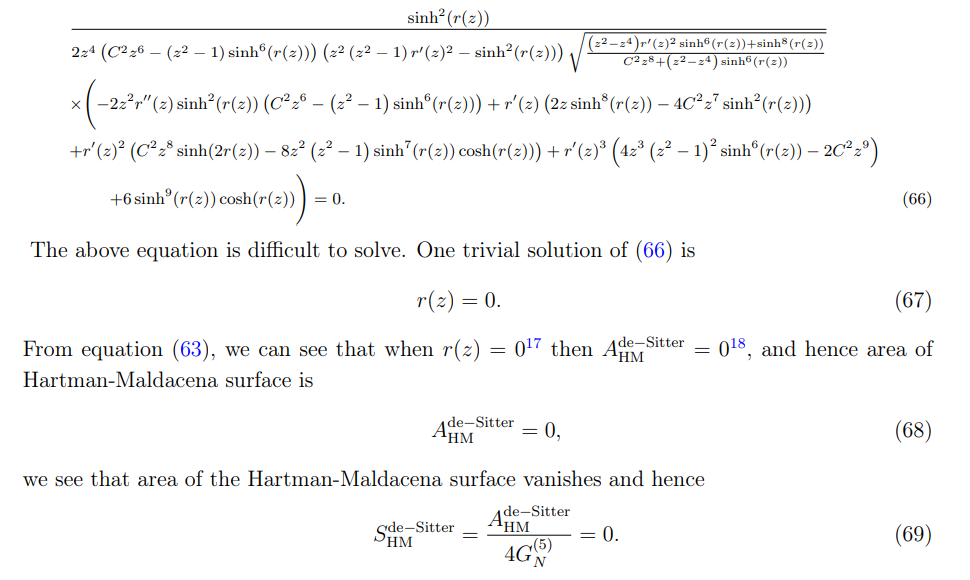
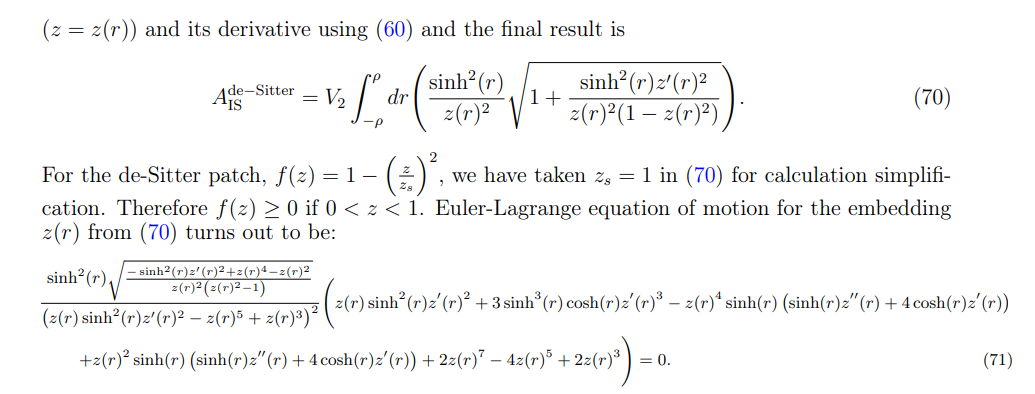
In general, it is not easy to solve the above equation. Interestingly, there is a z(r) = 1 solution to the above differential equation which is nothing but de-Sitter horizon assumed earlier(zs = 1) [19] and it satisfies the Neumann boundary condition on the branes and hence the solution for the cosmological island surface is

One can arrive at the same conclusion by requiring the well-defined variational principle of (70) and imposing Neumann boundary condition on the branes similar to the discussion in section 4.1 which requires
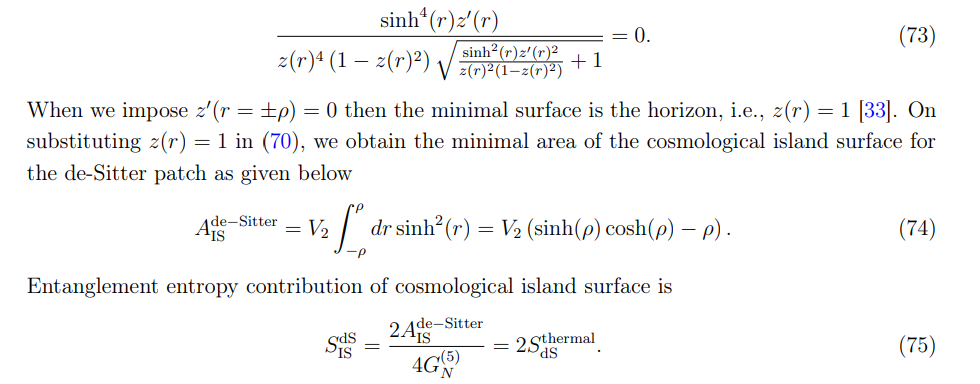
Additional numerical factor “2” is coming due to second cosmological island surface on thermofield double partner side (shown in Fig. 8). We get the Page curve of de-Sitter patch by plotting (69) and (75) from wedge holography. We will get a flat Page curve in this case similar to [33].
Let us summarize the results of this section. It was argued in [33, 35] that in wedge holography without DGP term, the black hole horizon is the only extremal surface and the HartmanMaldacena surface does not exist and hence one expects the flat page curve. We also see that when we compute the entanglement entropies of island surfaces of AdS, Schwarzschild, and deSitter black holes then minimal surfaces turn out to be horizons of the AdS or Schwarzschild or de-Sitterblack holes. As a curiosity, we computed entanglement entropies of Hartman-Maldacena surfaces for the parametrization r(z) and v(z) used in the literature and we found non-trivial linear time dependence for the AdS and Schwarzschild black holes whereas Hartman-Maldacena surface entanglement entropy turns out to be zero for the de-Sitter black hole. Therefore we obtain the flat Page curve for the de-Sitter black hole not for the AdS and Schwarzschild black holes due to the non-zero entanglement entropy of Hartman-Maldacena surfaces. The theme of the paper is not to discuss whether we get a flat Page curve or not. The paper aimed to construct a “multiverse” in Karch-Randall braneworld which we did in section 3 and check the formula given in (22). We saw in subsection 4.1 that (22) is giving consistent results.
Comment on the Wedge Holographic Realization of Schwarzschild de-Sitter Black Hole with Two Karch-Randall Branes: In subsection 4.2, we performed our computation of the Schwarzschild and de-Sitter patches separately. There is one more way by which we may get the Page curve of the Schwarzschild de-Sitter black hole. We summarize the idea below:
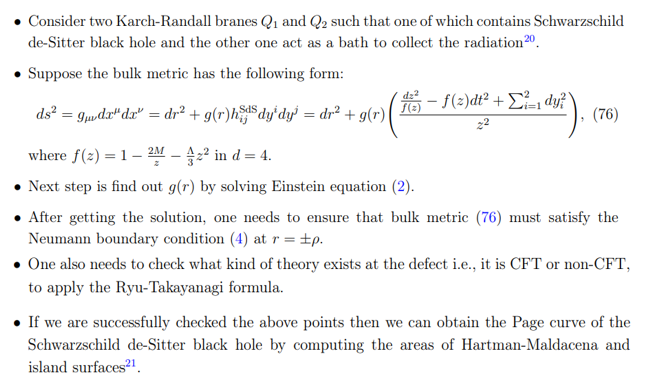
The above discussion is just a “mathematical idea”. Since we have three possible branes: Minkowski, de-Sitter and anti de-Sitter [55]. There is no brane with the induced metric defined in the open bracket of (76). Further, we have AdS/CFT correspondence or dS/CFT correspondence, or flat space holography. There is no such duality that states the duality between CFT and bulk which has the form of a Schwarzschild de-Sitter-like structure. There will be no defect description due to the aforementioned reason and hence no “intermediate description” of wedge holography. Therefore we conclude that one can model Schwarzschild de-Sitter black hole from wedge holography with two copies of wedge holography in such a way that one part defines Schwarzschild patch and the other part defines de-Sitter patch [22] .
[13] It was discussed in [33] that Neumann boundary condition on gravitating branes implies that Ryu-Takayanagi surface in the wedge holography is the black hole horizon. The same was also obtained in [35] by using inequality condition on the area of island surface. We obtained the same throughout the paper wherever we have discussed the entanglement entropy of island surfaces.
[14] We can show the same by following the steps given in detail from (63)-(67). But we have to replace the warp factor sinh(r(z)) by e r(z) .
[15] See the terms inside the open bracket of (57), there are terms with derivatives of z(r) and a particular combination (−8z(r) 2 + 4z(r) + 4z(r) 3 ) which vanishes for z(r) =
[16] We used zs = 1 only for the simplification of calculation. Since cosmological constant is very small and hence in reality zs >> 1 but some number which will not affect our qualitative results.
[17] Same solution r(z) = 0 also appeared in [35] in the computation of area of Hartman-Maldacena surface. See [33] for the similar solution, in our case embedding is r(z) whereas in [33], embedding is r(µ), µ being the angle.
[18] See [59] for the discussion of complexity of de-Sitter spaces.
[20] In this case, Hawking radiation will not be a suitable term because when Schwarzschild de-Sitter black hole as whole emits radiation then observer may not distinguish between Hawking radiation emitted by Schwarzschild patch and Gibbons-Hawking radiation emitted by de-Sitter patch [60].
[21] In this setup, the notion of “island” may become problematic because we will be talking about the island in the interior of Schwarzschild de-Sitter black hole. Since SdS black hole has two horizons, therefore it may cause trouble to say whether the “island” is located inside the black hole horizon or the de-Sitter horizon. Therefore it will be nice to follow the setup with two black holes and two baths. See [58] for non-holographic approach.
[22] See [58, 62] for non-holographic model.
This paper is available on arxiv under CC 4.0 license.